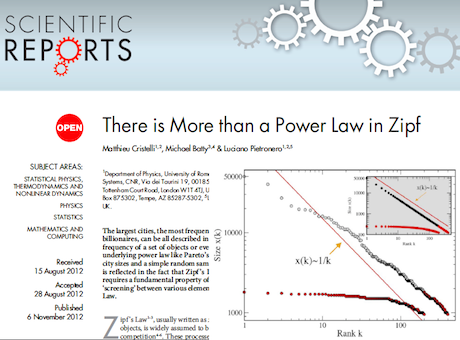
There is More Than a Power Law in Zipf

Posted on November 8, 2012 by Michael Batty

Check out our paper in Scientific Reports published on the 6th November. Click to Open Access, so no fear of breaking copyright by reading it ! What a surprise. We or rather a grant paid for it.   What we argue here is that if you examine a system of objects which follows some sort of skewed distribution that can be approximated by a power law, then if this system is incomplete in some way, then there is no way a power law can ever fit. For example, if you have a system of cities that excludes some that are clearly part of the system in terms of its evolution, a power law cannot result. We show that first by taking an income distribution which is generated from a pure power law, then break it into two and exclude the largest objects. Those left follow an entirely different distribution from the complete distribution. It is no longer scaling and no longer rank size in the Zipfian sense.

This means that if you have a system of cities that excludes some, a power law is also an incomplete representation of it. Pretty obvious really but surprising how little this issue has been discussed. A good example is the city size distribution in the  United States which excludes Canadian cities and in this case, we get something near a power law but not quite. Now to correct for this phenomenon, we show how you can sample from the distribution to base an approximation on a subset of values which mirrors the true distribution. Of course to do this, you have to assume the distribution is a power law and we never know whether or not this is the case. Catch 22 as ever. Nevertheless, our argument is completely general and it casts doubt on much of the work on fitting power laws to rank size and related distributions that has been carried out over the last 50 or more years. Food for thought at least. The idea that the system is incomplete in some way is much more general than power laws per se as it suggests that we need to pay attention to the system and figure out in advance how complete it is likely to be, or rather how complete the data we have is. We don’t really do this too much in our field. We should. Read On.

Posted in city size, Fractals, power laws, rank size, Scaling | Comments Off on There is More Than a Power Law in Zipf

Disruption in Large Transit Systems

Posted on October 12, 2012 by Michael Batty

Jon Reades and myself gave a talk at ECCS on how we are using our Oyster Card data to simulate disruptions to the London tube system. Added Richard Milton’s real time tube and bus data and presented our ideas at IFISC in Palma on 11th October. They made a nice show of this and if you click here you will get the online video which tells you much more about our project that I can tell you here.

Basically our project uses 6 months of RFID smart card like data where people use the tube and overground rail systems in London by swiping in and swiping out of stations thus making a journey from which the cost is deducted from the card. We have great flow data and we are able to examine several ways of disrupting the network – in turn disrupting the flows by closing stations and then figuring out what the impact if on other stations in the netowkr. We are searching really for locations – stations – which are particularly vulnerable to such disruptions which might be physical attacks like 7/7, train breakdowns, strikes on the line and so on. There is a lot to model in such problems and this post gives you some sense of how we are beginning to approach the project. Watch the powerpoint above or download the pdf by clicking here. Coming soon that is.

Posted in Big Data, Connectivity, Flows, Interactions, Smart Cities | Leave a comment

Research, Write, Type, Submit, Pass, and 30 Years Later …..

Posted on October 5, 2012 by Michael Batty

… chop the thesis up, scan it, and put it online for all those who should have been reading it this past three decades but couldn’t, wouldn’t, never knew it existed and so on. Or have never read a thesis in a university library where they are stored as most of us haven’t and don’t know where they are.

Growing in my mind and everyone else’s is the idea that we can scan all the old books and papers to make them available online. Google seem to have cornered the market in much of this but the open access movement is putting everything online anyway. A lot has been done but on a personal level, it is still quite painful. Recently we acquired a copier that let’s us scan papers and email them as pdf files to the author and this is so fast that it has opened up all sorts of intriguing possibilities.

So first in line was my PhD thesis from 1984, submitted late in life for a student, but long ago enough for it to be historic. Typed and then bound and submitted, never published but archived away on a shelf in the University of Wales library in central Cardiff  where it has been gathering dust ever since, I decided to give it a go. Two copies remained in my possession, bound in the usual way, so first I had to dissemble one of them and the picture above shows what we did. The printer who still binds our hard copies of Environment and Planning B, the journal I edit, can of course do it in reverse. Last night I scanned the entire dissembled thesis in about 15 minutes and assembled the pdf files into one, reduced them and popped them on to one of our servers.

So here it is for all to enjoy ! Click on the various links to download it. I doubt it will be a best seller but there is plenty in it about linear dynamics and urban models that is still relevant, I think. And maybe, just maybe someone will find something in it that inspires them to extend the state of the art in ways that we should have been doing this last 30 years ! Enjoy.

Posted in Entropy, Flows, Information, Urban Models | Leave a comment